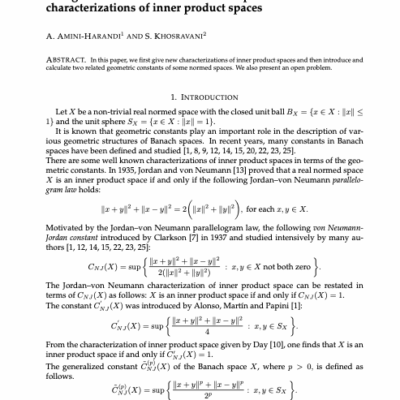
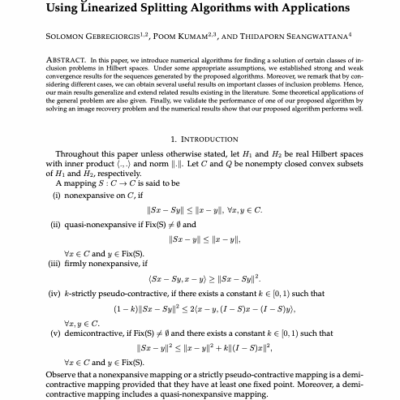
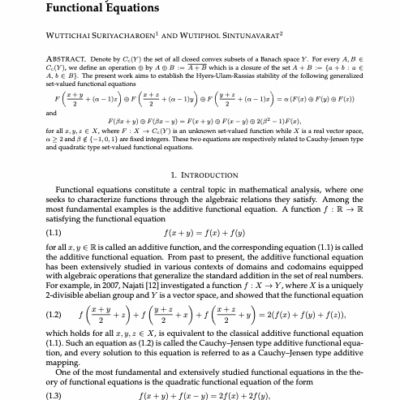
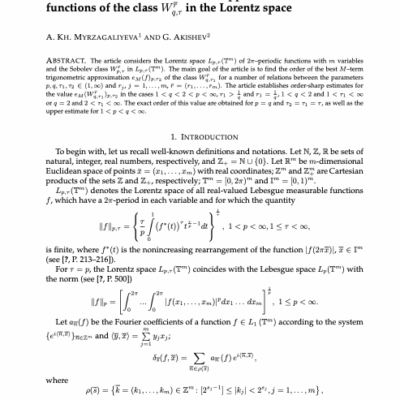
Let ![]() be the graded polynomial algebra over
be the graded polynomial algebra over ![]() where
where ![]() denotes the prime field of two elements. We investigate the Peterson hit problem for the polynomial algebra
denotes the prime field of two elements. We investigate the Peterson hit problem for the polynomial algebra ![]() viewed as a graded left module over the mod-
viewed as a graded left module over the mod-![]() Steenrod algebra,
Steenrod algebra, ![]() For
For ![]() this problem is still unsolved, even in the case of
this problem is still unsolved, even in the case of ![]() with the help of computers.
with the help of computers.
In this paper, we study the hit problem for the case ![]() in degree
in degree ![]() with
with ![]() an arbitrary non-negative integer. By considering
an arbitrary non-negative integer. By considering ![]() as a trivial
as a trivial ![]() -module, then the hit problem is equivalent to the problem of finding a basis of
-module, then the hit problem is equivalent to the problem of finding a basis of ![]() -graded vector space
-graded vector space ![]() The main goal of the current paper is to explicitly determine an admissible monomial basis of the
The main goal of the current paper is to explicitly determine an admissible monomial basis of the ![]() -graded vector space
-graded vector space ![]() in some degrees. At the same time, the behavior of the sixth Singer algebraic transfer in degree
in some degrees. At the same time, the behavior of the sixth Singer algebraic transfer in degree ![]() is also discussed at the end of this article. Here, the Singer algebraic transfer is a homomorphism from the homology of the mod-
is also discussed at the end of this article. Here, the Singer algebraic transfer is a homomorphism from the homology of the mod-![]() Steenrod algebra,
Steenrod algebra, ![]() to the subspace of
to the subspace of ![]() consisting of all the
consisting of all the ![]() -invariant classes of degree
-invariant classes of degree ![]()