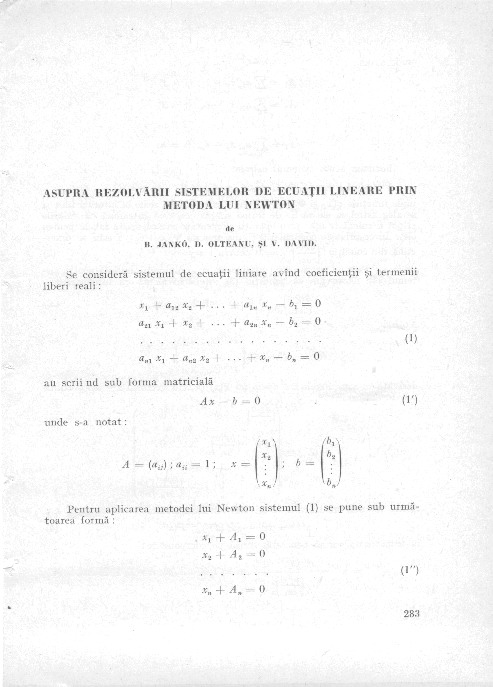
Asupra rezolvarii sistemelor de ecuatii liniare prin metoda lui Newton
Janko, B. ♦ Olteanu D. ♦ David B.
Abstract
Full PDF
Let (X,d)(X,d) be a complete metric space and let f:X→Xf:X→X be a self operator. In this paper we study the following two problems: Problem 1. Let ff be such that its fixed points set is a singleton, i.e., Ff={x∗}Ff={x∗}. Under which conditions the next implication does hold: ff is asymptotically regular ⇒⇒ ff is a Picard operator? Problem 2. Let ff be such that, Ff≠ϕFf≠ϕ. Under which conditions the following implication does hold: ff is asymptotically regular ⇒⇒ ff is a weakly Picard operator? The case of operators defined on a linear L∗L∗-space is also studied.