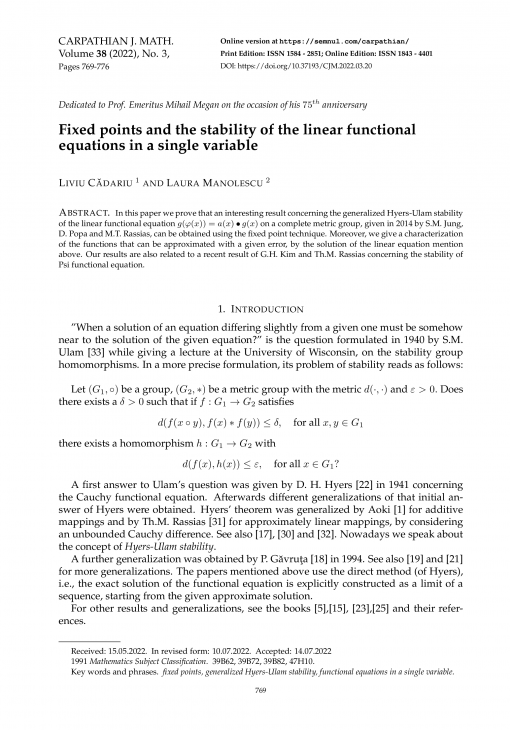
In this paper we prove that an interesting result concerning the generalized Hyers-Ulam stability of the linear functional equation 
on a complete metric group, given in 2014 by S.M. Jung, D. Popa and M.T. Rassias, can be obtained using the fixed point technique. Moreover, we give a characterization of the functions that can be approximated with a given error, by the solution of the linear equation mention above.
Our results are also related to a recent result of G.H. Kim and Th.M. Rassias concerning the stability of Psi functional equation.
Fixed points and the stability of the linear functional equations in a single variable
Cădariu, Liviu and Manolescu, Laura
Full PDF
carpathian_2022_38_3_769_776Additional Information
| Author(s) | Manolescu, Laura, Cădariu, Liviu |
|---|---|
| DOI | https://doi.org/10.37193/CJM.2022.03.20 |
Recently posted
-
An inertial method for solving the split equality fixed point problem with multiple output sets
-
Asymptotic modeling of non-linear viscopiezoelectric Kelvin-Voigt type plates via Trotter theory
-
Properties of isocompact spaces in topological groups
-
Two generalized cyclic projection algorithms for solving a class of the split feasibility problem in real Hilbert spaces
-
Global-fixed-point property of gyrogroup actions
-
On simple normal structure and best proximity points in reflexive Banach space
-
Unified Convergence Analysis of Certain At Least Fifth Order Methods
-
A set packing model for the Partition Coloring Problem
-
Cut-through connections of graphs
-
Positive solutions for multipoint boundary value problem of fractional differential equation with parameter