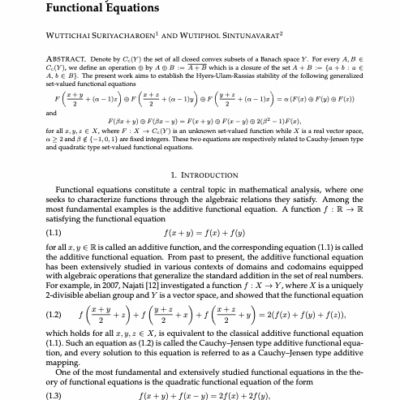
carpathian_2024_40_2_243_261In this work, we propose and study an inertial Krasnosel’skiĭ-Mann iterative algorithm with step-size parameters involving nonexpansive mapping to find a solution of a fixed point problem of a nonexpansive mapping in the frame work of Hilbert spaces. Strong convergence of the new proposed algorithm is proved under some useful properties of a nonexpansive mapping and inequalities on real Hilbert spaces together with the appropriate conditions of scalar controls without relying on the concept of viscosity methods. For the applications, we employ the obtained results to find a zero point of some monotone inclusion problems and then apply to solve image restoration problems. For representing the advantage of the new algorithm, the signal to noise ratio (SNR) with various types of blurring operators and some numerical experiments are presented to compare and illustrate the behavior of the new algorithm with numerical results of some previous existing algorithms.
