On a generalization of the Levin-May Theorem

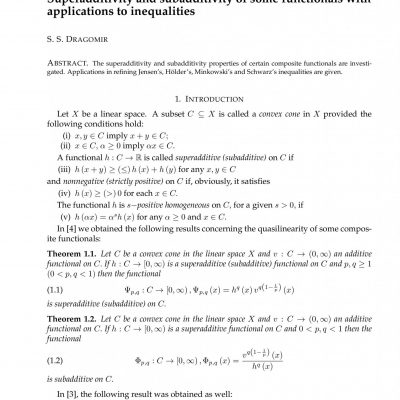
The paper discusses a distribution of the zeros of the polynomial

with respect to the unit circle. This problem is of theoretic as well as practical importance which motivated S. A. ~Levin and R. May to formulate a necessary and sufficient condition guaranteeing the location of all the zeros of inside the unit circle. We give a simple alternate proof of their criterion and, as the main result, present a complete list of all possible z  ero distributions of with respect to this circle.