On approximating fixed points of weak enriched contraction mappings via Kirk’s iterative algorithm in Banach spaces

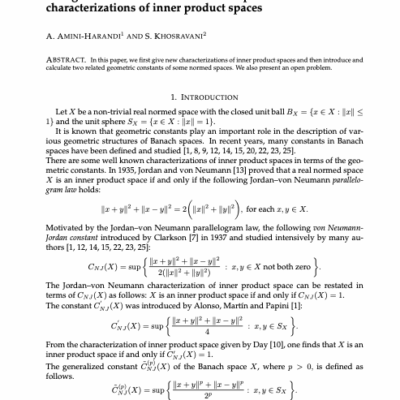
Recently Berinde and Păcurar [Approximating fixed points of enriched contractions in Banach spaces.  J. Fixed Point Theory Appl. 22 (2020), no. 2., 1–10], first introduced the idea of enriched contraction mappings and proved the existence of a fixed point of an enriched contraction mapping using the well-known fact that any fixed point of {the averaged mapping , where , is also a fixed point of the initial mapping }. In this work, we introduce the idea of weak enriched contraction mappings, and a new generalization of an averaged mapping called double averaged mapping. The first attempt is to prove the existence and uniqueness of the fixed point of a double averaged mapping associated with a weak enriched contraction mapping. Based on this result on Banach spaces, we give some sufficient conditions for the equality of all fixed points of a double averaged mapping and the set of all fixed points of a weak enriched contraction mapping. Moreover, our results show that an appropriate Kirk’s iterative algorithm can be used to approximate a fixed point of a weak enriched contraction mapping. An illustrative example for showing the efficiency of our results is given.