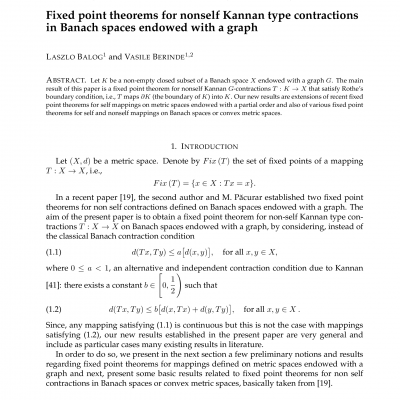
The main aim of the paper is to give the crossing number of join product ![]() for the wheel
for the wheel ![]() on six vertices, and
on six vertices, and ![]() consisting of
consisting of ![]() isolated vertices. In the proofs, it will be extend the idea of the minimum numbers of crossings between two different subgraphs from the family of subgraphs which do not cross the edges of the graph
isolated vertices. In the proofs, it will be extend the idea of the minimum numbers of crossings between two different subgraphs from the family of subgraphs which do not cross the edges of the graph ![]() onto the family of subgraphs that cross the edges of
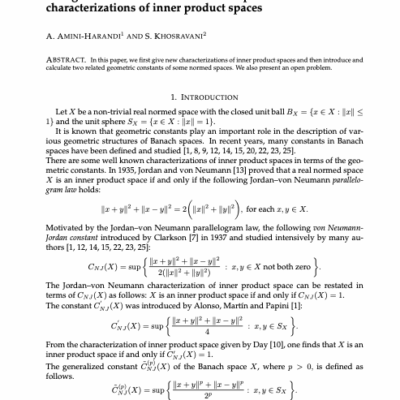
onto the family of subgraphs that cross the edges of ![]() at least twice. Further, we give a conjecture that the crossing number of
at least twice. Further, we give a conjecture that the crossing number of ![]() is equal to
is equal to ![]() for
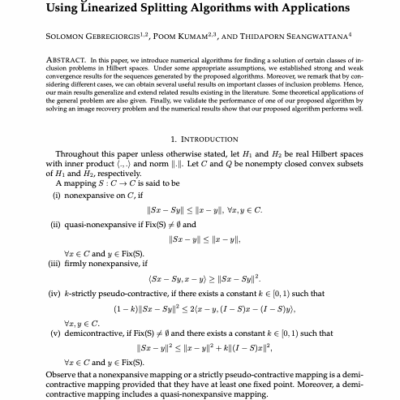
for ![]() at least three, and where the Zarankiewicz’s number
at least three, and where the Zarankiewicz’s number ![]() is defined for
is defined for ![]() . Recently, our conjecture was proved for the graphs
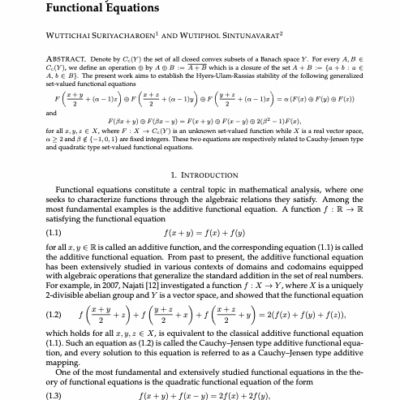
. Recently, our conjecture was proved for the graphs ![]() , for any
, for any ![]() , by Klešč et al., and also for
, by Klešč et al., and also for ![]() and
and ![]() due to the result by Klešč, Schr\”otter and by Staš, respectively. Clearly, the main result of the paper confirms the validity of this conjecture for the graph
due to the result by Klešč, Schr\”otter and by Staš, respectively. Clearly, the main result of the paper confirms the validity of this conjecture for the graph ![]() .
.