We prove an identity theorem for Gâteaux holomorphic functions on polygonally connected 
-open sets, which yields a very general maximum norm principle and a sublinear “max-min” principle. All results apply in particular to vector-valued functions which are holomorphic (in any sense that implies Gâteaux holomorphy) on domains in Hausdorff locally convex spaces.
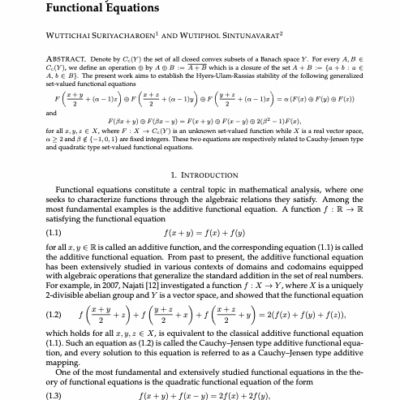
On the maximum modulus principle and the identity theorem in arbitrary dimension
Timofte, Vlad
Full PDF
carpathian_2022_38_2_517_522Additional Information
| Author(s) | Timofte, Vlad |
|---|---|
| DOI | https://doi.org/10.37193/CJM.2022.02.20 |
Recently posted
-
An inertial method for solving the split equality fixed point problem with multiple output sets
-
Asymptotic modeling of non-linear viscopiezoelectric Kelvin-Voigt type plates via Trotter theory
-
Properties of isocompact spaces in topological groups
-
Two generalized cyclic projection algorithms for solving a class of the split feasibility problem in real Hilbert spaces
-
Global-fixed-point property of gyrogroup actions
-
On simple normal structure and best proximity points in reflexive Banach space
-
Unified Convergence Analysis of Certain At Least Fifth Order Methods
-
A set packing model for the Partition Coloring Problem
-
Cut-through connections of graphs
-
Positive solutions for multipoint boundary value problem of fractional differential equation with parameter